2D Flow Around a Cylinder
This is a 2D time-dependent flow field. It has been simulated using the Gerris Flow Solver at a Reynolds number of 160. It is given on a uniform grid. Pre-computed streak line vector fields are available for this data set as well.
About the data set
The data set has been obtained from a numerical simulation using the Free Software Gerris Flow Solver. It is a 2D viscous flow around a simple solid boundary, i.e., the cylinder. Fluid is injected to the left of a channel bounded by solid walls with a slip boundary condition. Adaptive refinement is used based on both the vorticity and the gradient of a passive tracer, which is injected in the bottom half of the inlet. After an initial growth phase, the well-known von Kármán vortex street is formed. The cylinder itself is centered at x=y=0 with a diameter of 0.125 units.
This is a slightly modified version of an example that comes with the software. The sole modifications are the choice of a longer output time interval and the sampling onto a dense uniform grid. The von Kármán vortex street is fully developed in the chosen time interval.
Visualizations
The following figures show simple stream line visualizations using LIC. Streak line visualizations (including pre-computed streak line vector fields) are available for this data set as well. More information can be found in the paper: Streak Lines as Tangent Curves of a Derived Vector Field
 |
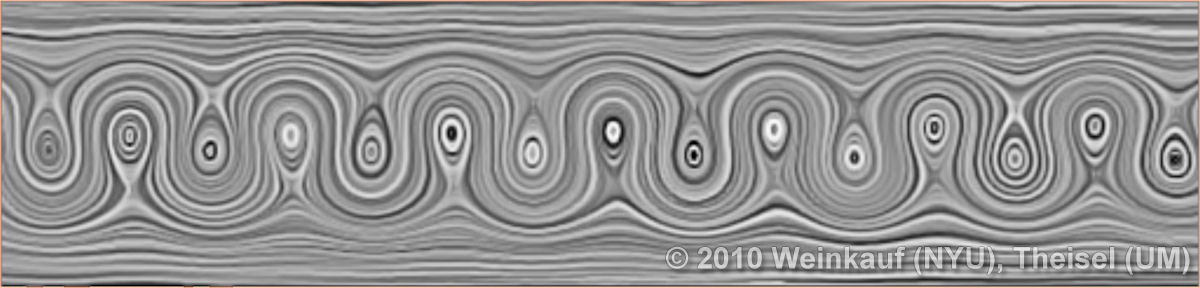 |
Left: stream lines in the original frame of reference. Right: Stream lines with removed ambient flow reveal the von Kármán vortex street.
Technical Details
The data set describes a 2D time-dependent flow field and therefore it consists of two components u,v specifying the direction of the flow at a certain location in space-time.
Resolution
The data set is given on a 3D uniform grid with the following specifications:
- Grid: 400 x 50 x 1001 (number of grid points in x,y,t-direction)
- Bounding Box: [-0.5, 7.5] x [-0.5, 0.5] x [15, 23] (extents in x,y,t-direction)
Data Format
It is written as a single file in AmiraMesh format.
Additional Files
In addition to the vector data, the surface of the cylinder itself is provided in the formats Stanford PLY, OpenInventor, and AmiraMesh HyperSurface. The first two formats can be read by almost all mesh processing programs, for example MeshLab.
Furthermore, the modified simulation settings are provided (cylinder.gfs).
How to Acknowledge
You are free to use this data set as long as you give proper acknowledgement. Please use a LaTeX snippet similar to the following:
This data set has been simulated by Tino Weinkauf \cite{weinkauf10c}
using the Free Software \emph{Gerris Flow Solver} \cite{gerrisflowsolver}.
with the following BibTeX entries:
@ARTICLE{weinkauf10c,
author = {T.~Weinkauf and H.~Theisel},
title = {Streak Lines as Tangent Curves of a Derived Vector Field},
journal = {IEEE Transactions on Visualization and Computer Graphics (Proceedings Visualization 2010)},
year = {2010},
volume = {16},
pages = {1225--1234},
number = {6},
month = {November - December},
abstract = {Characteristic curves of vector fields include stream, path, and streak
lines. Stream and path lines can be obtained by a simple vector field
integration of an autonomous ODE system, i.e., they can be described
as tangent curves of a vector field. This facilitates their mathematical
analysis including the extraction of core lines around which stream
or path lines exhibit swirling motion, or the computation of their
curvature for every point in the domain without actually integrating
them. Such a description of streak lines is not yet available, which
excludes them from most of the feature extraction and analysis tools
that have been developed in our community. In this paper, we develop
the first description of streak lines as tangent curves of a derived
vector field -- the streak line vector field -- and show how it can
be computed from the spatial and temporal gradients of the flow map,
i.e., a dense path line integration is required. We demonstrate the
high accuracy of our approach by comparing it to solutions where
the ground truth is analytically known and to solutions where the
ground truth has been obtained using the classic streak line computation.
Furthermore, we apply a number of feature extraction and analysis
tools to the new streak line vector field including the extraction
of cores of swirling streak lines and the computation of streak line
curvature fields. These first applications foreshadow the large variety
of possible future research directions based on our new mathematical
description of streak lines.},
keywords = {unsteady flow visualization, streak lines, streak surfaces, feature extraction},
url = {http://tinoweinkauf.net/}
}
@ARTICLE{gerrisflowsolver,
author = {S. Popinet},
title = {Free Computational Fluid Dynamics},
journal = {ClusterWorld},
year = {2004},
volume = {2},
number = {6},
url = {http://gfs.sf.net/}
}